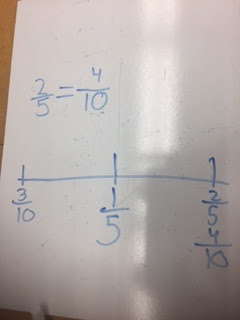Day 72: Coordinate Plane Quiz and Battleship

6th Grade Math Standards: 6.NS.6 Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. a. Recognize opposite signs of numbers as indicating locations on opposite sides of 0 on the number line; recognize that the opposite of the opposite of a number is the number itself, e.g., –(–3) = 3, and that 0 is its own opposite. b. Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane; recognize that when two ordered pairs differ only by signs, the locations of the points are related by reflections across one or both axes. c. Find and position integers and other rational numbers on a horizontal or vertical number line diagram; find and position pairs of integers and other rational numbers on a coordinate plane.

6.NS.8 Solve real-world and mathematical problems by graphing points in all four quadrants of the coordinate plane. Include use of coordinates and absolute value to find distances between points with the same first coordinate or the same second coordinate.

6.G.3 Draw polygons in the coordinate plane given coordinates for the vertices; use coordinates to find the length of a side joining points with the same first coordinate or the same second coordinate. Apply these techniques in the context of solving real-world and mathematical problems.

Objective: Locate a point in the coordinate plane; identify the four quadrants in the coordinate plane; reflect a point in the coordinate plane; create polygons in the coordinate plane; find the distance between two points in the coordinate plane

Agenda:

1. QSSQ 
2. Quiz on coordinate plane
3. Battleship brackets 

Assessment: Coordinate plane quiz

Glass Half-Full: Students in two of my three classes have been bothering me since the start of the unit to play Battleship. Today we did it. In a unique way compared to what I have done in the past, I set up brackets and had winning students play against other winning students and losing students play against other losing students. I explained the format before the quiz and they were all fired up. As a result of the students playing Battleship and not needing my direction or cueing them to task, I was able to grade the quizzes and call up individual students to clarify errors on this quiz or past assessments.

Regrets: There was a question that I had students find the distance between two points on a coordinate plane in which there was a trapezoid. We had gotten practice with finding the distance between two points, but always with peg boards or at least by looking at those points on a coordinate plane. I never left the students to find the answer with just giving them the coordinates as was the case on this quiz. Consequently, I passed out graph paper and told students to plot the trapezoid first to make finding the distance easier. I would like to remove this scaffold in the future though and will have to teach it in order to do so.

Day 71: Coordinate Plane Review

6th Grade Math Standards: 6.NS.6 Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. a. Recognize opposite signs of numbers as indicating locations on opposite sides of 0 on the number line; recognize that the opposite of the opposite of a number is the number itself, e.g., –(–3) = 3, and that 0 is its own opposite. b. Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane; recognize that when two ordered pairs differ only by signs, the locations of the points are related by reflections across one or both axes. c. Find and position integers and other rational numbers on a horizontal or vertical number line diagram; find and position pairs of integers and other rational numbers on a coordinate plane.

6.NS.8 Solve real-world and mathematical problems by graphing points in all four quadrants of the coordinate plane. Include use of coordinates and absolute value to find distances between points with the same first coordinate or the same second coordinate.

6.G.3 Draw polygons in the coordinate plane given coordinates for the vertices; use coordinates to find the length of a side joining points with the same first coordinate or the same second coordinate. Apply these techniques in the context of solving real-world and mathematical problems.

Objective: Locate a point in the coordinate plane; identify the four quadrants in the coordinate plane; reflect a point in the coordinate plane; create polygons in the coordinate plane; find the distance between two points in the coordinate plane

Agenda:

1. Which one doesn't belong? Number 5
2. QSSQ 
3. Review the coordinate plane reflections homework and exit ticket
4. Pepper
5. Try a couple more reflection problems (back of exit ticket was not done the previous day)
6. Study guide 
7. Peg boards to show what reflections are 

Assessment: The peg boards; back of exit tickets; pepper

Glass Half-Full: I lit a fire in the students regarding their lack of effort in the reflection problems. I was fine with them struggling on some of the theory questions on the homework, but the fact that they did not highlight demonstrated a weakness in their effort. Our quote of the day dealt with Elizabeth Spiegel who is known as the toughest and best chess coach in the country for students in the middle school grades. In the book How Children Succeed, she was extremely critical of a person on her team who took only two seconds to make a move. The quote and the process for learning reflections were well correlated and students got the message with my own coach like tone regarding their low efforts.

Regrets: The exit tickets demonstrated that students would struggle with the homework. Some of the homework questions would have been better utilized as classwork. For instance students were asked if points are reflected across both axes if the new points are (-x, -y) if they started out as (x, y). This was something completely over the heads of all but about three of my students. Yet in explaining it, students were interested in knowing more about this topic. It got to the point where one students asked me about reflecting across the line y = x to take a point and move to quadrants with one reflection (she of course did not use those exact words, but still...).

Day 70: Reflections in the Coordinate Plane

6th Grade Math Standards: 6.NS.6 Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. a. Recognize opposite signs of numbers as indicating locations on opposite sides of 0 on the number line; recognize that the opposite of the opposite of a number is the number itself, e.g., –(–3) = 3, and that 0 is its own opposite. b. Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane; recognize that when two ordered pairs differ only by signs, the locations of the points are related by reflections across one or both axes. c. Find and position integers and other rational numbers on a horizontal or vertical number line diagram; find and position pairs of integers and other rational numbers on a coordinate plane.

6.NS.8 Solve real-world and mathematical problems by graphing points in all four quadrants of the coordinate plane. Include use of coordinates and absolute value to find distances between points with the same first coordinate or the same second coordinate.

6.G.3 Draw polygons in the coordinate plane given coordinates for the vertices; use coordinates to find the length of a side joining points with the same first coordinate or the same second coordinate. Apply these techniques in the context of solving real-world and mathematical problems.

Objective: Reflect a point in the coordinate plane; determine the distance between two points that share one axis in a coordinate plane

Agenda:

1. Visual Pattern 
2. QSSQ 
3. Review homework
4. Use peg boards to see if students could create a rectangle and also count the distance between two points.
5. Reflections notes 
6. Use highlighters to find reflections of points in coordinate plane (exit ticket)
7. Pass out homework on reflections

Assessment: The peg boards was something I circumvented the room to ensure that students were recognizing where points went and how many spaces should be between points. I also circumvented the room to ensure students were highlighting as I asked on the exit ticket (many needed a reminder).

Glass Half-Full: Experience creates anticipation. I knew students would reflect points across the wrong axis and was able to jump on them when they did this for not highlighting which axis (or fence as I use in an analogy). Hopefully that will show up in their homework.

Regrets: This lesson was slightly rushed. I need to do a better job of bringing up reflections in the real world such as how the word ambulance is written or how a zit on one side of your face shows up on the other side of your face in the mirror. With a real world connection, there will be a higher interest level and investment from the students.

Day 69: Intro to Coordinate Plane

6th Grade Math Standards: 6.NS.8 Solve real-world and mathematical problems by graphing points in all four quadrants of the coordinate plane. Include use of coordinates and absolute value to find distances between points with the same first coordinate or the same second coordinate.

6.G.3 Draw polygons in the coordinate plane given coordinates for the vertices; use coordinates to find the length of a side joining points with the same first coordinate or the same second coordinate. Apply these techniques in the context of solving real-world and mathematical problems.

Objective: Locate a point in the coordinate plane; identify the quadrant of a point in the coordinate plane

Agenda:

1. Self-Assessment
2. QSSQ
3. Review Quiz
4. Provide students coordinate plane notes and graphic organizer
5. Practice
6. Homework

Assessment: I circumvented the room as students tried the last practice problems and homework problems on their own.

Glass Half-Full: The graphic organizer allows for me to do other things as students get the notes in partners. I put grades into the grade book in one class and made the initial plans to a weekly quiz two weeks down the line as a result of this in class. Students barely needed any instruction from me and if they did it was a four second answer.

Regrets: The students are really struggling with what is negative and positive. It's as if the integers unit did not happen. I wish that we had done the kinesthetic version of this lesson where the desks become grouped into different quadrants and students can get used to what negative is within the context of our "homemade coordinate plane."

Day 68: Integers Quiz

6th Grade Math Standards: 6.NS.6 Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. a. Recognize opposite signs of numbers as indicating locations on opposite sides of 0 on the number line; recognize that the opposite of the opposite of a number is the number itself, e.g., –(–3) = 3, and that 0 is its own opposite. b. Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane; recognize that when two ordered pairs differ only by signs, the locations of the points are related by reflections across one or both axes. c. Find and position integers and other rational numbers on a horizontal or vertical number line diagram; find and position pairs of integers and other rational numbers on a coordinate plane.

6.NS.7 Understand ordering and absolute value of rational numbers. a. Interpret statements of inequality as statements about the relative positions of two numbers on a number line diagram. For example, interpret –3 > –7 as a statement that –3 is located to the right of –7 on a number line oriented from left to right. b. Write, interpret, and explain statements of order for rational numbers in real-world contexts. For example, write –3o C > –7o C to express the fact that –3o C is warmer than –7o C. c. Understand the absolute value of a rational number as its distance from 0 on the number line; interpret absolute value as magnitude for a positive or negative quantity in a real-world situation. For example, for an account balance of –30 dollars, write |–30| = 30 to describe the size of the debt in dollars. d. Distinguish comparisons of absolute value from statements about order. For example, recognize that an account balance less than –30 dollars represents a debt greater than 30 dollars.

Objective: Order integers; Justify why an integer is bigger than another integer; define absolute value; evaluate an expression with absolute value; define integer

Agenda:

1. Jumpstart
2. QSSQ 
3. Review absolute value and comparing integers homework
4. Pepper
5. Integers study guide
6. Integers quiz 
7. Weekly Quiz 
8. Challenge problem 

Assessment:

Glass Half-Full: During pepper we were emphasizing how to find 10 percent of a number. This was a gap in student learning two days previous to this point in the year as evidenced by the quizzes we took on percentages. When students faced a question that interleaved the percentages standards on the integer quiz, they handled it with much more success than they had previously.

Regrets: I do not think I was explicit enough with students about a number further to the right on a number line being greater than a number to the left on the number line. Many students cited that they would rather owe three dollars than seven dollars when justifying that -3 is greater than -7 (which I allowed), but I think the number line understanding is more mathematically pleasing (for lack of a better term because I'm typing all of these recaps weeks after I actually taught them).

I also do not like students saying that -3 is greater because it is closer to zero. This rule only applies to negative numbers being compared to other negative numbers and students are failing to mention this within their explanation.

Day 67: Absolute Value and Ordering Integers

6th Grade Math Standards: 6.NS.6 Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. a. Recognize opposite signs of numbers as indicating locations on opposite sides of 0 on the number line; recognize that the opposite of the opposite of a number is the number itself, e.g., –(–3) = 3, and that 0 is its own opposite. b. Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane; recognize that when two ordered pairs differ only by signs, the locations of the points are related by reflections across one or both axes. c. Find and position integers and other rational numbers on a horizontal or vertical number line diagram; find and position pairs of integers and other rational numbers on a coordinate plane. 7.

6.NS.7 Understand ordering and absolute value of rational numbers. a. Interpret statements of inequality as statements about the relative positions of two numbers on a number line diagram. For example, interpret –3 > –7 as a statement that –3 is located to the right of –7 on a number line oriented from left to right. b. Write, interpret, and explain statements of order for rational numbers in real-world contexts. For example, write –3o C > –7o C to express the fact that –3o C is warmer than –7o C. c. Understand the absolute value of a rational number as its distance from 0 on the number line; interpret absolute value as magnitude for a positive or negative quantity in a real-world situation. For example, for an account balance of –30 dollars, write |–30| = 30 to describe the size of the debt in dollars. d. Distinguish comparisons of absolute value from statements about order. For example, recognize that an account balance less than –30 dollars represents a debt greater than 30 dollars.

Objective: Evaluate expressions with absolute value brackets; define what zero means in the context of a real world problem; order integers; analyze why one integer is greater than another integer

Agenda:

1. Self-Assessment
2. QSSQ 
3. Review the quiz 
4. Review the integers homework practice 
5. Introduce absolute value (Dunkin Donuts)
6. Students do absolute value practice independently
7. Skit with ordering integers
8. Comparing and ordering integer notes
9. Comparing and ordering integer practice

Assessment: At the end of each class, students were able to start homework assignments as I circumvented the room; as part of the self-assessments students stated whether they made simple mistakes or did not understand concepts from the quiz

Glass Half-Full: Despite the high number of items on the agenda, today's lessons had a good flow to them. Each concept can keep be explained and even analyzed quickly. When I told students they would have another quiz tomorrow, they were a little shocked since that would mean only two days between quizzes, but this was truly all that was necessary.

Regrets: The only problem with these lessons is that I do not cover ordering of rational numbers and really do not touch the term rational numbers in these lessons. Students had some difficulty when ordering all negative numbers, but once I pointed it out to them, they were quick to see this in future problems. Numbers such as -5.75 on a number line are a little more troublesome for many students though and I failed to really hit that today.

Day 66: Percentages Quiz & Integers Intro

6th Grade Math Standards: 6.NS.6 Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates. a. Recognize opposite signs of numbers as indicating locations on opposite sides of 0 on the number line; recognize that the opposite of the opposite of a number is the number itself, e.g., –(–3) = 3, and that 0 is its own opposite. b. Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane; recognize that when two ordered pairs differ only by signs, the locations of the points are related by reflections across one or both axes. c. Find and position integers and other rational numbers on a horizontal or vertical number line diagram; find and position pairs of integers and other rational numbers on a coordinate plane.

6.NS.7. Understand ordering and absolute value of rational numbers. a. Interpret statements of inequality as statements about the relative positions of two numbers on a number line diagram. For example, interpret –3 > –7 as a statement that –3 is located to the right of –7 on a number line oriented from left to right. b. Write, interpret, and explain statements of order for rational numbers in real-world contexts. For example, write –3o C > –7o C to express the fact that –3o C is warmer than –7o C. c. Understand the absolute value of a rational number as its distance from 0 on the number line; interpret absolute value as magnitude for a positive or negative quantity in a real-world situation. For example, for an account balance of –30 dollars, write |–30| = 30 to describe the size of the debt in dollars. d. Distinguish comparisons of absolute value from statements about order. For example, recognize that an account balance less than –30 dollars represents a debt greater than 30 dollars.

6.RP.3 Use ratio and rate reasoning to solve real-world and mathematical problems, e.g., by reasoning about tables of equivalent ratios, tape diagrams, double number line diagrams, or equations. a. Make tables of equivalent ratios relating quantities with whole-number measurements, find missing values in the tables, and plot the pairs of values on the coordinate plane. Use tables to compare ratios. b. Solve unit rate problems, including those involving unit pricing and constant speed. For example, if it took 7 hours to mow 4 lawns, then, at that rate, how many lawns could be mowed in 35 hours? At what rate were lawns being mowed? c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent. d. Use ratio reasoning to convert measurement units; manipulate and transform units appropriately when multiplying or dividing quantities.

Objective: Convert between percentages, decimals, and fractions; order percentages, decimals and fractions; find the part given whole; find the whole given part; use percentages to solve real world problems

Agenda:

1. QSSQ 
2. Percentages Quiz 
3. Work on WQ 
4. After Quiz Challenge
5. Pepper
6. An integer is all whole numbers, their opposites, and zero
7. Intro to Integers
8. Integers homework practice

Assessment: Integers notes were done with independent practice and the teacher circumventing the classroom; the quiz was assessed for a grade

Glass Half-Full: I think this is the right time of the year to teach one of the simpler concepts we have. Students do not have to make as many calculations with integers, absolute value, etc. in sixth grade. And at this time of the year (right before Christmas and New Year's), there are plenty of distractions so this is a good time to take it easier.

Regrets: There are still obvious fundamentals lacking in student's abilities to solve percent problems. Not that I'm not accountable for that, but I did send a note off to all parents and guardians about having their child be responsible for calculating the tip at a restaurant because these kids are at the point where they go to restaurants without supervision. Is the tip supposed to be random? Are they just going to Google it? Calculating a tip was something I learned very young, and it's a little disappointing to see the lack of experience my students have with this skill.

Day 65: Percentages Study Guide

6th Grade Math Standards: Use ratio and rate reasoning to solve real-world and mathematical problems, e.g., by reasoning about tables of equivalent ratios, tape diagrams, double number line diagrams, or equations. a. Make tables of equivalent ratios relating quantities with whole-number measurements, find missing values in the tables, and plot the pairs of values on the coordinate plane. Use tables to compare ratios. b. Solve unit rate problems, including those involving unit pricing and constant speed. For example, if it took 7 hours to mow 4 lawns, then, at that rate, how many lawns could be mowed in 35 hours? At what rate were lawns being mowed? c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent. d. Use ratio reasoning to convert measurement units; manipulate and transform units appropriately when multiplying or dividing quantities.

Objective: Convert between percentages, decimals, and fractions; order percentages, decimals and fractions; find the part given whole; find the whole given part; use percentages to solve real world problems

Agenda:

1. Visual Pattern #27
2. QSSQ 
3. Skipped reviewing homework and opted instead for my own couple of problems I made up on the spot. 
4. Study Guide 
5. Stations: 99 Restaurant Tip, other practice material 

Assessment: Students worked in groups on the stations and I sat in on two specific stations. The 99 restaurant station (which challenged them) and then a percent problem that forced students to consider the thousandths place as it was converted to a decimal were the places I put myself. The study guide was done individually or in partners and then reviewed for all students.

Glass Half-Full: I think that problems escalated in difficulty as the days progressed in this unit. Each day logically built on the preceding day and at the same time all students were challenged without being pushed past the point of giving up. Kind of the goal of teaching, right?

Regrets: Some of the station stuff was impossible to review because of the pace of the group activities and my availability and necessary instruction at the two stations that I needed to visit.

Day 64: Part Given Whole

6th Grade Math Standards: 6.RP.3 Use ratio and rate reasoning to solve real-world and mathematical problems, e.g., by reasoning about tables of equivalent ratios, tape diagrams, double number line diagrams, or equations. a. Make tables of equivalent ratios relating quantities with whole-number measurements, find missing values in the tables, and plot the pairs of values on the coordinate plane. Use tables to compare ratios. b. Solve unit rate problems, including those involving unit pricing and constant speed. For example, if it took 7 hours to mow 4 lawns, then, at that rate, how many lawns could be mowed in 35 hours? At what rate were lawns being mowed? c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent. d. Use ratio reasoning to convert measurement units; manipulate and transform units appropriately when multiplying or dividing quantities.

Objective: Find the part given the whole amount; find the whole given the part; find the percent given the part and the whole

Agenda:

1. Open Middle
2. QSSQ 
3. Review of percent of a number homework 
4. Proportion frayer model
5. Part Given whole notes
6. Part given whole practice 

Assessment: Students did some problems from the notes on their own as I circumvented and other students helped.

Glass Half-Full: The Open Middle problem was something students struggled with despite the fact that Robert Kaplinsky calls it a fourth grade problem. I decided not to go over the answer in any classes and hang onto the problem as a bonus for the quiz.

Regrets: I got lazy and did not interleave well at all on these notes. Students got used to setting up proportions, but never had to think about what number was a part and what number was a whole because the part was always the number that was given. If I could have even added one problem in which the whole was given (percent of a number) it would have forced students to think more about how the problem was written. These problems are an issue of literacy as much as math once the basic understanding of what a percent is met.

Day 63: 20 Percent Off or 20 Dollars Off?

6th Grade Math Standards: 6.RP.3 Use ratio and rate reasoning to solve real-world and mathematical problems, e.g., by reasoning about tables of equivalent ratios, tape diagrams, double number line diagrams, or equations. a. Make tables of equivalent ratios relating quantities with whole-number measurements, find missing values in the tables, and plot the pairs of values on the coordinate plane. Use tables to compare ratios. b. Solve unit rate problems, including those involving unit pricing and constant speed. For example, if it took 7 hours to mow 4 lawns, then, at that rate, how many lawns could be mowed in 35 hours? At what rate were lawns being mowed? c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent. d. Use ratio reasoning to convert measurement units; manipulate and transform units appropriately when multiplying or dividing quantities.

Objective: Determine if 20% off or $20 off is a better deal

Agenda:

1. Self Assessment
2. QSSQ
3. Review the Quiz
4. My Favorite No (20% of 64)
5. 20% off or $20 off from Dan Meyer 
6. Homework Practice

Assessment: I circumvented the room as students worked on the homework and the notes. There was also the self-assessment sheet that students got after the quiz. One of the sixty students could find the answer to the my favorite no question. That student used multiplication, so I worked with that student on the ten percent rule.

Glass Half-Full: This was probably the fourth time I've used Dan Meyer's Dualing Discount activity to teach how to find the percent of a number. It was probably the best that I have done because I let the students know ahead of time not to shout out ideas to enhance the likelihood that many students would be discovering that sometimes 20% was better and sometimes $20 off was better.

Regrets: This lesson does take more than one would think just looking at the agenda and especially the dueling discount problems. I did not use all of Meyer's problems, and did not need to either. My frustration was that we could not have a discussion or a a calculation surrounding at what point does the price of the item not matter regardless of the coupon. Perhaps I could speed this up by giving students four more examples (not have them do the calculation) and then give them more time to analyze what was happening.

Day 62: Fraction, Percentages, and Decimals Quiz

6th Grade Math Standards: 6.RP.3c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent.

Objective: Convert between fractions, percentages, and decimals; compare and order fractions, decimals ,and percentages

Agenda:

1. Quick study guide review (especially interleaving problem)
2. QSSQ 
3. Take the quiz
4. Fix the weekly quiz 
5. Find two factors that have a product of 1,000,000
6. At the start of the next class pass out notes on comparing and ordering fractions, percentages, and decimals but I had students work in groups rather than do it as a lecture. The format worked well in the disciplined classes, but as much in the classes that were hyperactive. 
7. Did page 135 #25-27 for homework. 

Assessment: Circumvented the room during number six. The quiz and weekly quiz were corrected by me.

Glass Half-Full: I'm happy that I only gave three homework problems. No need for more as I can tell who is doing well and who needs remediation from there.

Regrets: On the quiz most students struggled with making 3/8 a percentage. It could have been better highlighted as an area of concern coming into today. Not to say that the concept was not reviewed, but we should have been a star next to the concept of percentages with decimals.

Day 61: Final Quiz Prep

6th Grade Math Standards: 6.RP.3c c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent.

Objective: Convert fractions, percentages, and decimals

Question of the Day: Is there any difference between 0.42 and .42?

Agenda:

1. Visual Pattern #34
2. QSSQ 
3. Review HW (#13-15 and the back) & Pepper
4. My Favorite No. Convert 0.3 into a percent. Convert 7% into a decimal.
5. Practice of decimal and percentage conversion
6. Study guide of converting between fractions, percentages, and fractions

Assessment: Circumventing the room during items three, five, and six of the agenda. During item two students simply brought their sticky notes up to me.

Glass Half-Full: The pace of this allowed for a good deal of feedback. I had students in work in partners on item number five in the agenda and I just circumvented the classroom. It was very apparent that the only difficulty involved one digit numbers or mixed numbers. Anything two digits the students had down. This was interesting because I resisted the temptation of telling students to slide the decimal two places. Instead I wildly praised students for writing each as a fraction out of 100 before converting.

I had students do the study guide as if it were the actual quiz. Inevitably I did give away answers and help them along, but it had a similar feel and the study guide was very much on par with the quiz.

Regrets: The homework from the night before should have had all the whole numbers changed from 2 to 1 in the four multiple choice options. I was the one that created that particular question, so score one for the math students.

Day 60: Percent Defined

6th Grade Math Standards: 6.RP.3c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent.

Objective: Convert fractions to percentages; convert percentages and decimals

Agenda:

1. Open Middle (third grade problem) 
2. QSSQ 
3. Pepper
4. Practice with fraction and decimal conversion
5. Percentage definition 
6. Converting between fractions, decimals and percentages notes
7. Fractions, decimals and percentages practice

Assessment: I circumvented the room as students worked out solutions to the problems. I really tried to sell students to write out the fraction before finding the decimal equivalent of a fraction. Single digit numbers are frequently a misunderstood concept because of a lack of place value knowledge and the ignorance of what the term percentage really means.

Glass Half-Full: Despite the third grade label of the warm up problem, the students were challenged and required to persevere. It was a problem that brought out students that do not normally shine and brought down those that normally do.

Regrets: I did not really do a good job of connecting the importance of this lesson to the real world. In past years, I take a box score from a sporting game, but I forgot today and also part of me said that the kids that hate sports probably hate me.

Day 59: Decimal & Fraction Conversion

6th Grade Math Standards: 6.RP.3c c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent.

Objective: Convert from fractions to decimals

Agenda:

1. Self-Assessment
2. QSSQ 
3. Review Test
4. My Favorite No. Convert 5/6 to a decimal. Find your grade.
5. Notes on conversion from decimal to fraction and then fraction to decimal
6. Exit Tickets. 

Assessment: The my favorite no was telling. In one class nobody could convert five-sixths to a decimal. One student said 0.83, but without the repitin bar. All year myself and the social studies teacher have been putting the grades in fraction form. It turns out the students would have practically no clue to know what they actually got for a grade (see picture). They did much better with the grade after the feedback of five-sixths was given.



There was also the exit ticket.

Glass Half-Full: We had a curriculum day earlier in the week. The focus for the whole year has been on writing. The cry from the math department is that we did not need writing as much. Now I'm typically of an open mind, so I went into today's meeting willing to learn, think, and be persuaded.

Ultimately the conclusion I reached was (as the common core sort of states) that I am a writing teacher. With that thought in mind, I really tried to focus on the exit ticket from a writing perspective. I wanted students to nail the math by telling a fifth grade student how to convert (see objective), but also to use solid transition words in describing the process. What I got was a mixed bag. That was not the important part. The important part was incorporating professional development into my lesson immediately after receiving professional development.

Regrets: The writing prompt should state that the directions on conversions are for a fifth grader and that I'm focused on the transition words, but I explained this orally. Like I said, focusing beyond the math in their writing is a new thing for me.

Day 58: Fraction Test

6th Grade Math Standards: Interpret and compute quotients of fractions, and solve word problems involving division of fractions by fractions, e.g., by using visual fraction models and equations to represent the problem. For example, create a story context for (2/3) ÷ (3/4) and use a visual fraction model to show the quotient; use the relationship between multiplication and division to explain that (2/3) ÷ (3/4) = 8/9 because 3/4 of 8/9 is 2/3. (In general, (a/b) ÷ (c/d) = ad/bc .) How much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 3/4-cup servings are in 2/3 of a cup of yogurt? How wide is a rectangular strip of land with length 3/4 mi and area 1/2 square mi?

Objective: Divide fractions to find a quotient; locate a fraction between two numerators of a like denominator that are next to each other on the number line (find what's between 10/3 and 11/3); multiply fractions to find a product; add fractions to find a sum; subtract fractions to find a difference

Agenda:

1. QSSQ
2. Fractions Test
3. Work on WQ 
4. Challenge Problems

Assessment: The test.

Glass Half-Full: The operations were done on problems one through eight and if that was the entire test grades would have been much higher. Kids can carry out the algorithm. Not surprisingly it's the higher order stuff such as application in the real world and finding holes between 10/3 and 11/3 where we struggled.

Regrets: The assessment included an interleaving question of 46 divided by 5. Students were instructed to find it using decimals, but I never reviewed this as part of the study guide the day prior, so I was not as stringent as the other math teachers probably were when students gave an answer of 9 and 1/5.

I also feel like this test is not as high up Bloom's Taxonomy as it could be.

Day 57: Who Wants to Be a Millionaire?

6th Grade Math Standards: 6.NS.1 Interpret and compute quotients of fractions, and solve word problems involving division of fractions by fractions, e.g., by using visual fraction models and equations to represent the problem. For example, create a story context for (2/3) ÷ (3/4) and use a visual fraction model to show the quotient; use the relationship between multiplication and division to explain that (2/3) ÷ (3/4) = 8/9 because 3/4 of 8/9 is 2/3. (In general, (a/b) ÷ (c/d) = ad/bc .) How much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 3/4-cup servings are in 2/3 of a cup of yogurt? How wide is a rectangular strip of land with length 3/4 mi and area 1/2 square mi?

Objective: Divide fractions in order to find a quotient; Place a fraction with a different denominators between two like fractions with numerators that are one apart on a number line (what fraction is between 11/3 and 12/3?)

Question of the Day: "Why is it that 3 and 4 will bring the same product and least common multiple, but 9 and 6 do not have the same product and least common multiple?"

Agenda:

1. Determine what fraction is between 11/3 and 12/3
2. Visual Pattern #32
3. QSSQ 
4. Review Homework and Pepper
5. Who Wants to Be a Millionaire (second class). All 13 questions are going to appear on the test (students do not know this) 

Assessment: The students used individual marker boards during Who Wants to Be a Millionaire; I circumvented the room for homework and pepper

Glass Half-Full: The way the timing worked out in this lesson could not have been much better. The routine of steps one through four combined with the novelty of step five in the second class was the right balance for engagement and learning to happen across many types of learners. Everything done was assessable and gave students an indication of where they were proficient and where they were lacking skills (needed reteaching or simply to cross their T's).

Regrets: I could not do all 13 questions on Who Wants to Be a Millionaire as a result of reteaching as I found student misconceptions. Ultimately, I think I would have been better off if I had strategically placed a question with each operation as well as the objective of locating fractions between number lines back to back. What ended up happening was that one class never needed to find a fraction between two like fractions and another did nothing but find a fraction between two like fractions as a result of me trying to compensate for my mistake in the first lesson. Just need to find a balance between the two and could do so by skipping around on Who Wants to Be a Millionaire rather than doing each question one by one. Students these days do not really remember how Who Wants to Be a Millionaire is supposed to be played anyway.

Day 56: Fraction Stations

6th Grade Math Standards: 6.NS.1 Interpret and compute quotients of fractions, and solve word problems involving division of fractions by fractions, e.g., by using visual fraction models and equations to represent the problem. For example, create a story context for (2/3) ÷ (3/4) and use a visual fraction model to show the quotient; use the relationship between multiplication and division to explain that (2/3) ÷ (3/4) = 8/9 because 3/4 of 8/9 is 2/3. (In general, (a/b) ÷ (c/d) = ad/bc .) How much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 3/4-cup servings are in 2/3 of a cup of yogurt? How wide is a rectangular strip of land with length 3/4 mi and area 1/2 square mi?

Objective: Interpret the relationship between fractions with unlike denominators on a number line.

Question: How is a fraction like 10 and 25/24 going to be fixed?

Agenda:

1. Estimation 180 (Lights)
2. QSSQ
3. Stations (word problem strategies, number line, stop and think, peanut butter blossoms, WQ) 
4. Exit Ticket (using clickers)
5. Start the homework 

Assessment: I stayed at one group of desks and focused on the number line concept that was listed in the objective.

Glass Half-Full: The clickers exit ticket was new to this year and really helped alleviate some of my concern regarding an issue that is a chronic red x when students take the test. They are overwhelmed by the number of steps involved in these problems so I tried to keep it really simple by saying get everyone to speak the same language. In other words make them all improper or mixed and then make them all have the same denominator and then reevaluate the question.

Regrets: Not even checking in on the groups in the other stations was a huge issue because it meant that they were unaccountable. For students that lack self-discipline this meant that they essentially got nothing done. Coming off of Thanksgiving break and getting back into fractions after a five day layoff, that meant that they might be lacking the skills they need to have.

Day 55: The Brain Bowl

For the second straight year before Thanksgiving we brought the entire sixth grade together to do a "so far year in review" trivia challenge. Every student is given a TurningPoint Clicker and then given multiple choice questions from all four core subjects (science, social studies, ELA, and math) as well as pop culture and random trivia questions (who played college sports for example). The clickers keep track of score automatically for us, so the students enjoy the competitive aspect of playing for their homeroom and for their core team (either Alcott or Hawthorne in our school). After about 30 minutes, two students are then chosen from each of the ten homerooms to compete in a final round. Where we continue to give multiple choice questions and give those twenty students exposure and make all of the things that they have learned and worked hard to practice worth knowing - even if they asked, "When are we going to use this in the real world?" 

I like this activity on this day for two reasons. First of all, units are being wrapped up or should be put on hold if they are not being wrapped up at this time of the year. To give an assessment on this day is hard because the classes are shortened with a half-day. If classes were to be held as if it were business as usual, the students would be unlikely to bring the same level of focus we typically see and some students are also already gone to visit grandma in Connecticut. Second, it gives the students a sense of school pride and a positive affiliation with school. This is a marathon and the enjoyment of this experience is building the appreciation of each curriculum for the students. Did we get closer to conquering the common core, getting a perfect score on PARCC, enrolling in Harvard, curing cancer, and teaching the future first president to achieve world peace and plant wheat crops on Mars? Not quite. What we did do was give students a fun memory from the year and also got a decent formative assessment for our troubles. 

Day 54: Dividing by Multiplying by the Reciprocal

6th Grade Math Standards: 6.NS.1 Interpret and compute quotients of fractions, and solve word problems involving division of fractions by fractions, e.g., by using visual fraction models and equations to represent the problem. For example, create a story context for (2/3) ÷ (3/4) and use a visual fraction model to show the quotient; use the relationship between multiplication and division to explain that (2/3) ÷ (3/4) = 8/9 because 3/4 of 8/9 is 2/3. (In general, (a/b) ÷ (c/d) = ad/bc .) How much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 3/4-cup servings are in 2/3 of a cup of yogurt? How wide is a rectangular strip of land with length 3/4 mi and area 1/2 square mi?

Objective: Divide fractions by multiplying by the reciprocal of the divisor

Agenda:

1. Open Middle find a quotient of 1/20 
2. QSSQ
3. Review the homework
4. Reciprocals
5. Show students the difference between 6 divided by 2 and half of 6
6. Dividing by Multiplying by the reciprocal practice 

Assessment: I had students stand up when they tried multiplying by the reciprocal problems on their own; checking the homework

Glass Half-Full: The open middle problem was solved by the students, but not me. I gave up after five minutes and checked the solution to see if it was as hard as I was making it. I couldn't do it without using a whole number or improper fraction cause my brain was on Thanksgiving. When I saw the answers, I saw that I might not be qualified to teach mathematics since I gave up and since it was very very easy. When the students were able to do the problem with pretty high efficiency, it was confirmed that I should be thankful that I have a job because at times they could definitely take the mic from me.

Regrets: When students stood up, they had the correct answer, but were still finding a common denominator. Is there anything wrong with that? To me, this is one of those situations where maybe adding a tool to the toolbox is not a big deal.

Link: The most fun cities. Just for the record I've been to 7 of the best 10 and 4 of the best 5.

Day 53: Dividing Fractions with a Common Denominator

6th Grade Math Standards: 6.NS.1 Interpret and compute quotients of fractions, and solve word problems involving division of fractions by fractions, e.g., by using visual fraction models and equations to represent the problem. For example, create a story context for (2/3) ÷ (3/4) and use a visual fraction model to show the quotient; use the relationship between multiplication and division to explain that (2/3) ÷ (3/4) = 8/9 because 3/4 of 8/9 is 2/3. (In general, (a/b) ÷ (c/d) = ad/bc .) How much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 3/4-cup servings are in 2/3 of a cup of yogurt? How wide is a rectangular strip of land with length 3/4 mi and area 1/2 square mi?

Objective: Divide fractions using a common denominator

Agenda:

1. Visual Pattern #27
2. QSSQ 
3. Division of Fractions Intro. You and three friends get a pizza (I display a pizza on the board). Then I tell kids to look at the back wall to see what's different. When they turn around, I've changed the board to show that one slice is missing and your brother took it. They fell for the oldest trick in the book. Now how are we going to split the pizza with 7 slices (7/8 of a pie) among 4 people?
4. Think, pair, share
5. Review how to divide fractions by finding a common denominator
6. Notes including a number line 
7. Independent practice and homework

Assessment:

Glass Half-Full: I found this helpful link about dividing fractions from Republic of Math about 20 minutes before school and wanted to incorporate it somehow into the lesson. I thought it was a useful of thinking about the problems and technically the standard does use the term visual fraction model. I doubt most students found it helpful, but there were probably a few, and I'm teaching "what I'm supposed to."

I have never taught students by making them find a common denominator first. The thought occurred to me in the moment when students were solving the activator problem with the pizza that most of them were in essence trying to either split the pizza into thirty-seconds or making sure each person got 1.75 slices. I don't think that students were making any connection to multiplying by a reciprocal. That was only brought about as a result of memorization. As the above link does delve into the students could eventually learn about multiplication of the reciprocal through self-discovery, but it's usually not the first maneuver students go to. Plus when we start talking about integrating the other operations, it's less for students to be confused by.
Just find a stupid common denominator. Even if you multiply. I don't care. Do it.

Regrets: I was vague as to whether students needed to find the number of slices per person or the fraction of the whole pizza per person. The two questions are different, so it would be best to ask both on the same slide. More students determined 1.75 slices per person than 7/32 of a pizza per person.

Link: Good way to practice coordinate plane and celebrate Black Friday from Robert Kaplinsky.

Day 52: Why 4 and 1/3 Times 2/5 Isn't 4 and 2/15

6th Grade Math Standards: 6.NS.1 Interpret and compute quotients of fractions, and solve word problems involving division of fractions by fractions, e.g., by using visual fraction models and equations to represent the problem. For example, create a story context for (2/3) ÷ (3/4) and use a visual fraction model to show the quotient; use the relationship between multiplication and division to explain that (2/3) ÷ (3/4) = 8/9 because 3/4 of 8/9 is 2/3. (In general, (a/b) ÷ (c/d) = ad/bc .) How much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 3/4-cup servings are in 2/3 of a cup of yogurt? How wide is a rectangular strip of land with length 3/4 mi and area 1/2 square mi?

Objective: Analyze the products of fraction problems in a mathematical context; find products in a real world context through multiplying fractions or using equivalent ratios

Agenda:

1. Which one doesn't belong?
2. QSSQ 
3. Review the homework
4. Exit Ticket on why 4 and 1/3 times 2/5 does not equal 4 and 2/15
5. Mashed Potato Recipes from Yummy Math

Assessment: The homework was assessed to see students comfort level in cross reducing. Some students were on the fence as to how cross reducing would make life simpler and not more complicated. There was the exit ticket (the subject of the amount of liquid in the glass) and the mashed potato recipes were collected by me to see where students were on old concepts integrated with new concepts.

Glass Half-Full: About 83% of the students had something logical to say about the exit ticket. I think it helped that it was connected to the question of the day as well.

Regrets: The mashed potato exercise was good, but there was no time to review the results. I even gave them the answer to problem one. Somehow we have to do a better job of time management because it would have been good to review for the whole crew after collecting the work and seeing the various misconceptions and multiple points of entry for those that did solve.

 Here the student triples the ingredients when the number of people to serve was not tripled.

Here the student correctly changes the ingredient, but the denominators in each picture are different.

Link: Many of the ideas in this blog post about 16 Ideas for Student Projects Using Google Docs, Slides, and Forms I was familiar with, but I thought a cool add on for my curriculum was to have students create their own form and have classmates answer it when we do statistical questions.

Day 51: Multiplying Fractions

6th Grade Math Standards: 6.NS.1 Interpret and compute quotients of fractions, and solve word problems involving division of fractions by fractions, e.g., by using visual fraction models and equations to represent the problem. For example, create a story context for (2/3) ÷ (3/4) and use a visual fraction model to show the quotient; use the relationship between multiplication and division to explain that (2/3) ÷ (3/4) = 8/9 because 3/4 of 8/9 is 2/3. (In general, (a/b) ÷ (c/d) = ad/bc .) How much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 3/4-cup servings are in 2/3 of a cup of yogurt? How wide is a rectangular strip of land with length 3/4 mi and area 1/2 square mi?

6.G.2 Find the volume of a right rectangular prism with fractional edge lengths by packing it with unit cubes of the appropriate unit fraction edge lengths, and show that the volume is the same as would be found by multiplying the edge lengths of the prism. Apply the formulas V = lwh and V = bh to find volumes of right rectangular prisms with fractional edge lengths in the context of solving real-world and mathematical problems.

Question of the Day: Tom Brday throws 8 touchdowns for every three interceptions. What is the ratio of interceptions to touchdowns?
Objective: Multiply fractions by fractions to find a product; multiply fractions by whole numbers to find a product; multiply mixed fractions to find a product

Agenda:

1. Self-Assessment from the addition and subtraction quiz
2. QSSQ
3. Review the Quiz
4. My Favorite No 
5. Pepper
6. Notes
7. Clickers (exit ticket)
8. Homework started in class

Assessment: I had the students multiple 1/3 by 2/5 to see what they already knew about multiplication of fractions. In one of my classes 11 of 18 students answered 2.

During the notes, students were consistently asked to try on their own before I showed them what to do and I circumvented the room at this time. There was a popular answer of 4 and 1/3 times 2/5 being 4 and 2/15. That was such an issue that it is going to become the question of the day for tomorrow. 

The clickers were only used for one class. I had a two step problem that required students to add and subtract fractions as well as multiply them. 

Glass Half-Full: As the 6th grade standards above indicate, there is nothing that is directly saying that students need to multiply fractions. It is a fifth grade standard. They do have to build upon their knowledge of multiplying though in order to find volumes and make sense of dividing fractions. So the novice in me would say let's just go right to division. The novice in me died a few years ago. When 11/18 of students answer a question wrong, there is a fraction problem (pun intended). Today's lesson was necessary, and hopefully impacted the students recognition of how to carry out the algorithm.

Regrets: The notes are designed to show students why a fraction times a fraction cannot equal two or anything greater than one. It was put in there, but once the robots - I mean students - start to multiply the numerator by the numerator and the denominator by the denominator, they lose site of this concept.

Link: Lost at School was a valuable read for me (although it took me two months to finish because I'm a horrible reader at busy times) in terms of classroom discipline. I think it confirmed many things for me and also showed me that repeated disciplinary actions are cause to change the way you see a problem in the classroom.

Day 50: Fractions Quiz

6th Grade Math Standards: 3.NF.3, 4.NF.2, 5.NF.1

Objective: Add and subtract fractions with like and unlike denominators; apply fraction operations in a real-world context

Agenda:

1. Marker Boards Collaboration
2. QSSQ
3. Take the Quiz
4. Work on Weekly Quiz, do retakes, or read

Assessment: The marker boards and the quiz

I had students each do a problem that forced them to borrow (if that was the method they were comfortable with) to get a difference with unlike denominators. Each student in a group of four did one problem like the two below.

When done they added their four differences. Again using whatever method they were comfortable with. Here one group is using improper fractions and another is using mixed numbers (incorrectly). 

If they got the answer wrong of the sum of all four problems I just said wrong. I tried to stay away from explaining and left it up to the group to correct until they had what is seen below. 

Glass Half-Full: Typically I give the quiz in the first 50 minutes of class and if there are students who need to finish, they can work on it in the second 50 minutes. Today I knew the quiz would not take as long. As a result we did not start the class with the quiz. Each kid was given their own marker board and told to do four separate subtraction problems with unlike denominators in which they would need to borrow (if borrowing was their preferred method of solving). Then when each student got their difference they found a sum of their four answers.  I had to say almost nothing after explaining the task and students collaboratively worked out their errors. The whole task took roughly 30 minutes per class. It left me feeling like the students were prepared for the quiz.

Regrets: I think that some students are taking too long on quizzes. The fact that we needed over an hour to do this type of quiz is alarming. I promote persistence and Einstein's quote of "It's not that I'm so smart, I just stay with the problem longer." I also have to have my students prepare to take PARCC.

Link: Article for the National  Council of Teachers of Mathematics on 13 "rules" that may not be helping our students.

Day 49: Unlike Denominators & Borrowing

6th Grade Math Standards: 3.NF.3 Explain equivalence of fractions in special cases, and compare fractions by reasoning about their size. a. Understand two fractions as equivalent (equal) if they are the same size, or the same point on a number line. b. Recognize and generate simple equivalent fractions, e.g., 1/2 = 2/4, 4/6 = 2/3. Explain why the fractions are equivalent, e.g., by using a visual fraction model. c. Express whole numbers as fractions, and recognize fractions that are equivalent to whole numbers. Examples: Express 3 in the form 3 = 3/1; recognize that 6/1 = 6; locate 4/4 and 1 at the same point of a number line diagram. d. Compare two fractions with the same numerator or the same denominator by reasoning about their size. Recognize that comparisons are valid only when the two fractions refer to the same whole. Record the results of comparisons with the symbols >, =, or <, and justify the conclusions, e.g., by using a visual fraction model.

4.NF.2 Compare two fractions with different numerators and different denominators, e.g., by creating common denominators or numerators, or by comparing to a benchmark fraction such as 1/2. Recognize that comparisons are valid only when the two fractions refer to the same whole. Record the results of comparisons with symbols >, =, or <, and justify the conclusions, e.g., by using a visual fraction model

5.NF.1 Add and subtract fractions with unlike denominators (including mixed numbers) by replacing given fractions with equivalent fractions in such a way as to produce an equivalent sum or difference of fractions with like denominators. For example, 2/3 + 5/4 = 8/12 + 15/12 = 23/12 . (In general, a/b + c/d = (ad + bc) /bd.)

Objective: Add fractions with like and unlike denominators; Find the difference in a mixed number problem such that the fractional part of the number being subtracted by is smaller than the fractional part that is subtracting from the bigger number

Agenda:

1. Open Middle Equivalent Fractions 
2. Quote, Star Student, Question
3. Study Guide in Partners
4. Review of Study Guide
5. 5 Minutes of Pepper
6. Independent Study Guide

Assessment: I was circumventing the room throughout the study guide. As students struggled with borrowing, I would continue to provide them with borrowing types of questions until they had successfully met the objective.

Glass Half-Full: For being a 4th grade open middle question, the warm up problem definitely made us sweat. That being said, all of my students were able to access the problem and try it. They just did not immediately come to a conclusion about its solution. In one class, a few of the students that did answer it were students that have had their share of struggles in math this year. Meanwhile students that are typically asking to be challenged or need to be challenged were still working it out. It was kind of a nice self-esteem boost for these students.

Regrets: Not having computer access hurt this lesson a little. If students could check their answer themselves or at least plug their answers into a computer in which I could oversee everything it would have been more efficient for giving feedback.

Link: I think Twitter makes sense too.

Day 48: Adding and Subtracting Unlike Fractions

6th Grade Math Standards: 4.NF.3 Understand a fraction a/b with a > 1 as a sum of fractions 1/b. a. Understand addition and subtraction of fractions as joining and separating parts referring to the same whole. b. Decompose a fraction into a sum of fractions with the same denominator in more than one way, recording each decomposition by an equation. Justify decompositions, e.g., by using a visual fraction model. Examples: 3/8 = 1/8 + 1/8 + 1/8 ; 3/8 = 1/8 + 2/8 ; 21/8 = 1 + 1 + 1/8 = 8/8 + 8/8 + 1/8. c. Add and subtract mixed numbers with like denominators, e.g., by replacing each mixed number with an equivalent fraction, and/or by using properties of operations and the relationship between addition and subtraction. d. Solve word problems involving addition and subtraction of fractions referring to the same whole and having like denominators, e.g., by using visual fraction models and equations to represent the problem.

5.NF.1 Add and subtract fractions with unlike denominators (including mixed numbers) by replacing given fractions with equivalent fractions in such a way as to produce an equivalent sum or difference of fractions with like denominators. For example, 2/3 + 5/4 = 8/12 + 15/12 = 23/12 . (In general, a/b + c/d = (ad + bc) /bd.)
5.NF.2. Solve word problems involving addition and subtraction of fractions referring to the same whole, including cases of unlike denominators, e.g., by using visual fraction models or equations to represent the problem. Use benchmark fractions and number sense of fractions to estimate mentally and assess the reasonableness of answers. For example, recognize an incorrect result 2/5 + 1/2 = 3/7, by observing that 3/7 < 1/2 .

Quote of the Day: "If I had an hour to solve a problem, I'd spend the 55 minutes thinking about it and the last 5 minutes solving it." - Albert Einstein

Question of the Day (from students): Is it possible to have a mixed improper fraction?

Objective: Add and subtract fractions

Agenda:

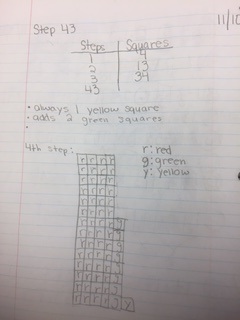
1. Visual Pattern #23
2. QSSQ 
3. Review Homework (#6, #8, #11, #16). These problems either involved mixed improper fractions or whole numbers subtracted by a fraction
4. My favorite no 11 and 3/5 minus 6 and 4/5.
5. Notes on my favorite no
6. My favorite no 11 and 4/5 minus 6 and 3/4
7. Notes on my favorite no
8. Marker boards to practice other problems that forced students to find common denominators

Assessment: The marker boards was an effective assessment today. It is much quicker for me to assess if students are writing on marker boards because the writing is bigger. The students are also a tick more engaged.

The my favorite no problems were also assessed as every student showed me their index cards. Most of them tried to say that the first problem's solution was 5 and 1/5 even though 3/5 minus 4/5 is negative. They gave a thumbs up to notify me that they were able to recognize this mistake when we reviewed.

In the second MFN problem, students for the most part knew to get a common denominator. The most common mistake I saw was that they failed to change the numerator after the denominators were changed.

On the homework, I consistently saw students leave answers as 2 and 9/8 or when they were asked to subtract 1 and 2/7 from 2 they got 1 and 2/7 for a difference instead of 5/7.

Glass Half-Full: It was great to diagnose the mistakes and why they were mistakes today. Having said that, I'm on a personal day on Monday and have a long weekend with Veteran's Day before we meet again as a class. Old habits and ways of thinking have a hard time of dying quickly, so it will be interesting to see what students carry over from today until we meet again in five days.

The other great part of the class today was the connection between the quote of the day and the Visual Pattern. Students were unable to get the answer immediately in all of my classes, but some of them did eventually crack the code.

To keep this in perspective, this picture was taken from a student that two months ago would have a blank stare at a visual pattern problem. It is unbelievable to see some of the transformations in the problem solving processes with these students - even if they are not all solving the problems.

Regrets: We rushed reviewing the homework problems and I still believe most of the students who could not do 2 - 1 and 2/7 for homework still cannot do it now. This will undoubtedly be part of my lesson on Tuesday.

Link: I liked this Tweet from Mark Chubb who asks what is a better question. What is closer to one on a number 4/5 or 5/4? Or 4/5 is closer than 5/4 than 1. Show why using a number line.

Day 47: Fraction Introduction

6th Grade Math Standards: 3.NF.3 Explain equivalence of fractions in special cases, and compare fractions by reasoning about their size. a. Understand two fractions as equivalent (equal) if they are the same size, or the same point on a number line. b. Recognize and generate simple equivalent fractions, e.g., 1/2 = 2/4, 4/6 = 2/3. Explain why the fractions are equivalent, e.g., by using a visual fraction model. c. Express whole numbers as fractions, and recognize fractions that are equivalent to whole numbers. Examples: Express 3 in the form 3 = 3/1; recognize that 6/1 = 6; locate 4/4 and 1 at the same point of a number line diagram. d. Compare two fractions with the same numerator or the same denominator by reasoning about their size. Recognize that comparisons are valid only when the two fractions refer to the same whole. Record the results of comparisons with the symbols >, =, or <, and justify the conclusions, e.g., by using a visual fraction model

Objective: Convert improper and mixed fractions

Agenda:

1. Self Assessment checklist from the test
2. Review the Ratio Test
3. QSSQ 
4. Fraction Notes
5. Hershey Bar
6. Adding and subtracting fractions with like denominators

Assessment: I tried to flip the classroom the day before this lesson by having students complete a Google Form after watching my video. About half of my students completed it.

Glass Half-Full: We defined the different types of fractions and what exactly a fraction is. Getting these core pieces is critical to making estimates and checking the work in future lessons with the four operations.

Regrets: This was a very easy lesson to create a pre-assessment for and I essentially did it with the Google Drive, but then I never bothered to differentiate the lesson. As the standards indicate above, this is a third grade skill. That being said 35% of students did not know how to convert between mixed and improper fractions, so it was worth the time to review for that crew, but the rest of the class could have been held to higher standards or given a harder task than the notes which they really already master.

Link: I follow Eric Sheninger on Twitter and I came across a nice Tweet from something he wrote back in August about a solution to negative people. Don't complain and if you do complain present two solutions to solve your problem or issue. He stole it from Jon Gordon's book No Complaining Rule. It's on my short list of must reads as I've also read The Energy Bus and Training Camp by the same author and gotten things out of those books.

Day 46 Ratios Test

6th Grade Math Standards: 6.RP.1 Understand the concept of a ratio and use ratio language to describe a ratio relationship between two quantities. For example, “The ratio of wings to beaks in the bird house at the zoo was 2:1, because for every 2 wings there was 1 beak.” “For every vote candidate A received, candidate C received nearly three votes.”
6.RP.2. Understand the concept of a unit rate a/b associated with a ratio a:b with b ≠ 0, and use rate language in the context of a ratio relationship. For example, “This recipe has a ratio of 3 cups of flour to 4 cups of sugar, so there is ¾ cup of flour for each cup of sugar.” “We paid $75 for 15 hamburgers, which is a rate of $5 per hamburger.” 29
6.RP.3. Use ratio and rate reasoning to solve real-world and mathematical problems, e.g., by reasoning about tables of equivalent ratios, tape diagrams, double number line diagrams, or equations. a. Make tables of equivalent ratios relating quantities with whole-number measurements, find missing values in the tables, and plot the pairs of values on the coordinate plane. Use tables to compare ratios. b. Solve unit rate problems, including those involving unit pricing and constant speed. For example, if it took 7 hours to mow 4 lawns, then, at that rate, how many lawns could be mowed in 35 hours? At what rate were lawns being mowed? c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent. d. Use ratio reasoning to convert measurement units; manipulate and transform units appropriately when multiplying or dividing quantities

Objective: Write a ratio three ways in a word problem context; find equivalent ratios by getting a unit rate; solve problems with ratio reasoning; apply a tape diagram to solve for an equivalent ratio; apply a double number line to solve ratio problems; find the least common multiple; compare two different prices

Agenda:

1. Quote, Star Student, Question
2. Take the Test
3. Retake any previous assessments from this year
4. 5, 4, 3, 2, 1 challenge (get to 20 through 30)
5. Read a book

Assessment: The test results were about what I would expect. This was a test that featured many concepts and covered almost all of the ratio common core standards, so there were definitely students with gaps. Class averages were in the high 70s. What will be interesting to see is how these gaps get filled moving forward. In general, ratios do not always correlate with future topics such as algebra and statistics, so I may explore getting these concepts in.

Here is an example of students getting two different answers (bottom is correct, top is incorrect) by doing the problem two different ways. I have used this problem for three years now and like the depth it forces students to use when they solve it multiple ways. 

Glass Half-Full: On part three of the agenda, students were allowed to retake any test or quiz from earlier this year. Students have folders in my room which show me which questions that they have answered wrong on any prior test or quiz we have had at this point in the year. It's not always the most efficient way to differentiate (I would love to rely on software instead), but it does give students the opportunity to increase their grade and me the opportunity to reteach and motivate students to continue learning the skills that they lack. Students were retaking quizzes from September and October (not the exact questions, but similar ones) and answering them well. This was encouraging to me because I do not think there's anyway that these skills can be classified as simply memory activating their brains. They were truly capable of performing the skill and this is a sign of mastery.

Regrets: Many students approach me during a test to sneak an answer out of me. I very rarely will comply. I wonder what research says about providing assistance during tests. My personal view which was influenced by Rick Wormeli is that mastery is not mastery until it is independent.

Link: That quiz was a resource I just heard about on Twitter. Not very fancy, but seems easy to use and a good place for remediation, pre-assessment, and even homework.

Day 45: Ratios Study Guide

6th Grade Math Standards: 6.RP.1 Understand the concept of a ratio and use ratio language to describe a ratio relationship between two quantities. For example, “The ratio of wings to beaks in the bird house at the zoo was 2:1, because for every 2 wings there was 1 beak.” “For every vote candidate A received, candidate C received nearly three votes.”
6.RP.2. Understand the concept of a unit rate a/b associated with a ratio a:b with b ≠ 0, and use rate language in the context of a ratio relationship. For example, “This recipe has a ratio of 3 cups of flour to 4 cups of sugar, so there is ¾ cup of flour for each cup of sugar.” “We paid $75 for 15 hamburgers, which is a rate of $5 per hamburger.” 29
6.RP.3. Use ratio and rate reasoning to solve real-world and mathematical problems, e.g., by reasoning about tables of equivalent ratios, tape diagrams, double number line diagrams, or equations. a. Make tables of equivalent ratios relating quantities with whole-number measurements, find missing values in the tables, and plot the pairs of values on the coordinate plane. Use tables to compare ratios. b. Solve unit rate problems, including those involving unit pricing and constant speed. For example, if it took 7 hours to mow 4 lawns, then, at that rate, how many lawns could be mowed in 35 hours? At what rate were lawns being mowed? c. Find a percent of a quantity as a rate per 100 (e.g., 30% of a quantity means 30/100 times the quantity); solve problems involving finding the whole, given a part and the percent. d. Use ratio reasoning to convert measurement units; manipulate and transform units appropriately when multiplying or dividing quantities

Objective: Write a ratio three ways in a word problem context; find equivalent ratios by getting a unit rate; solve problems with ratio reasoning; apply a tape diagram to solve for an equivalent ratio; apply a double number line to solve ratio problems; find the least common multiple; compare two different prices

Agenda:

1. Which one doesn't belong (Number 11 from Mrs. Morgan)
2. QSSQ 
3. Collect WQs and Review HW 
4. Start the study guides 
5. Review the study guides

Assessment: Circumventing the room as students worked on the study guide. The study guide is definitely longer than a full class. In the future I can cut problems out of the study guide. Most notably numbers five, six and any two of numbers fourteen through sixteen. It is a busy test with almost the entire ratio standard incorporated (with the exception of percentages using proportions).

Glass Half-Full: A colleague who teaches ELA came to observe my classroom last block and he was very complimentary of the way in which I have the students use highlighters to find the information that is important in a word problem. As he put it, treating word problems as close reading activities helps reinforce literacy skills and problem solving skills.

We also tried something spontaneous that the ELA teacher liked. All of the students were encouraged to send me a picture of them studying. The test was coming after a weekend and the study guide was given on a Friday. Experience has taught me that testing on a Monday inevitably leads to worse results. There are a number of factors that are likely to cause this - most notably that memory is not as strong so this was something worth a shot.

Regrets: Aside from what was already mentioned with eliminating problems, I feel as though students would also benefit from an answer key readily available to them on this study guide due to the high number of problems that they were asked to do. I also firmly believe students do not take advantage of this accommodation regularly because they are unaware of it and have never been taught with answer keys before. The security of knowing that they did the right process and got the right answer does not have to be exclusive to me walking around. Unfortunately we do not have Chromebooks on a 1:1 ratio (no pun intended given the content we were focused on) and I do not like to have to photocopy this many answer keys. I could have students check their phones, but my room gets awful service. Excuses are lovely but I can certainly navigate around all of these excuses.

Link: Warren Buffet drove people in a trolley to get out to vote. It was great to see the unified spirit of the country to just vote in so many instances (without actually following that with vote for ____). Having said that, Buffet of course did openly endorse Hilary.