Question of the Day: How do we do the distributive property?
Regular Math Lesson Objective: Solve expressions with exponents
Regular Math Sequence:
- Self-Assessments completed as students reviewed their quizzes in groups. I had to really prompt groups to work together to help students that got problems wrong. Rather than saying out loud "go over this with your groups" I literally had to go to a kid in a group and ask them what the correct answer for a certain problem was. When they were lacking confidence I looked at someone else in their group of four who had the correct answer and said "ask them." I wish this was not the case, but for some reason (maybe it's insecurity maybe it's lack of executive function) this sort of prompting is necessary.
- QSSQ
- I reviewed whatever was left for going over quizzes after groups had a chance to review together
- Exponent exploration to find exponents with positive bases and negative exponents. I derived this from an Illustrations discussion about how students arrived at negative exponents. I messed up question four slightly and have since fix it to say that the two bases are opposites not the two exponents. I really enjoyed this packet because it served as a great way to activate prior knowledge for the lower students especially and it was clear that the high students benefitted because nobody that I could see correctly labeled 2 to the 0 power, 2 to the negative 1, and so on. Students were saying of course that 2 to the 0 was 1.
- Exponent exit ticket. Most students were able to find 8 to the third power (as I would hope) and -8 to the third power (I was not as sure on that one). Nobody could find 8 to the negative third. I told them to really think about this one and challenged them throughout the day by stating that nobody had been able to do it. Most of the answers I saw were either -512 or 512. There were some other creative answers.
Question of the Day: How do we know if there will only be one solution in an absolute value equation?
Honors Math Lesson Objective: Graph and write inequalities
Honors Math Sequence:
- Pass back quizzes and do a self assessment.
- Review the quizzes (it only took 40 minutes!)
- Exploration with inequalities (Big Ideas generated)
The review of the quizzes felt therapeutic. I had many students get below a grade that is their expectation for themselves, so I told them things were rainbows and puppy dogs, and told them to focus on the math.
The inequalities work was much briefer than I would have liked, but we did at least get to graph a couple and write a couple. It seems like the students feel comfortable with the whole process, but I'll begin tomorrow with what was supposed to be today's exit ticket.



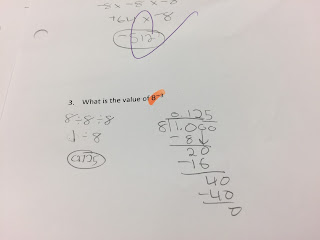
No comments:
Post a Comment